How to Calculate Ratios (With Examples To Do It Easily)
Updated March 19, 2024
Ratios are mathematical constructs for assessing the relationship between two or more numerical values.
They serve to evaluate either absolute quantities and magnitudes or to gauge relative portions within a larger context.
While ratios can be computed and expressed in various formats, the fundamental principles governing their utilization remain consistent across all methods.
In this article, we explain how to calculate ratios easily.
What Are Ratios?
A ratio is a mathematical term used to describe how much of one thing there is in comparison to another thing.
How to Calculate a Ratio
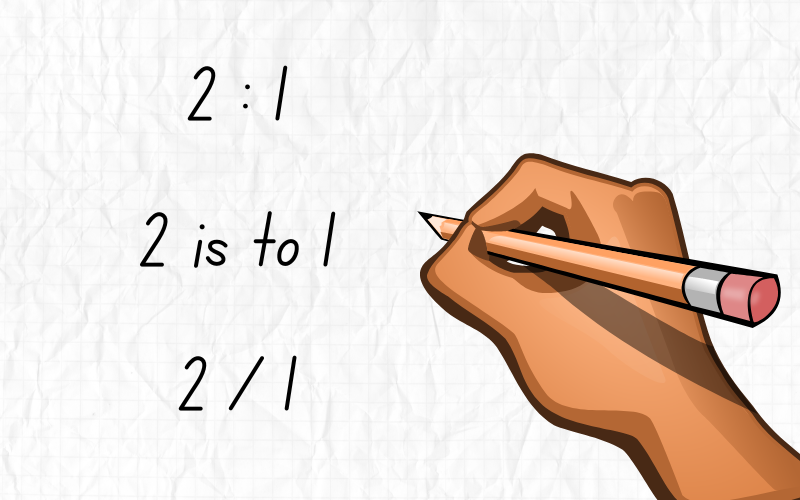
Ratios are usually written in the following formats:
- 2:1
- 2 to 1
- 2/1
Used in mathematics and everyday life, you may have come across ratios without knowing it – for example in scale drawings or models, in baking and cooking, and even when converting currency for a holiday abroad.
Ratios are useful when you need to know how much of one thing there needs to be in comparison with another thing.
Knowing how to find a ratio is easier when you know how they work, and how a ratio might be presented in different scenarios.
Examples on Ratio Formula
Example 1
In a bag of 20 sweets, the ratio of blue to pink might be 2:3
The use of ratio in this example will inform us that there would be 8 blue sweets and 12 pink sweets. (This question and the way to work it out is detailed below).
Example 2
If you are making a cake, and you require 3 cups of flour and 2 cups of sugar to make enough to feed 10 people, then you can express that as the ratio 3:2.
Prepare for Any Job Assessment Test with JobTestPrep
To scale the ingredients up to feed 20 people (to double the recipe size) you need to double the ingredients – so you would need 6 cups of flour and 4 of sugar (or 6:4).
Understanding how to calculate a ratio will make it easier for you to deal with these everyday scenarios.
How To Do Ratios – Sample Questions and Their Solutions
Understanding how to work out ratios is an important skill and can be particularly useful when applying for jobs where a good understanding of mathematics is required.
It is a good idea to revise skills like this before taking numerical reasoning or other math-based aptitude tests.
Prepare for Any Job Assessment Test with JobTestPrep
Here are the key ratio skills that you need to master. See the given explanation for a full breakdown on how to find the answer:
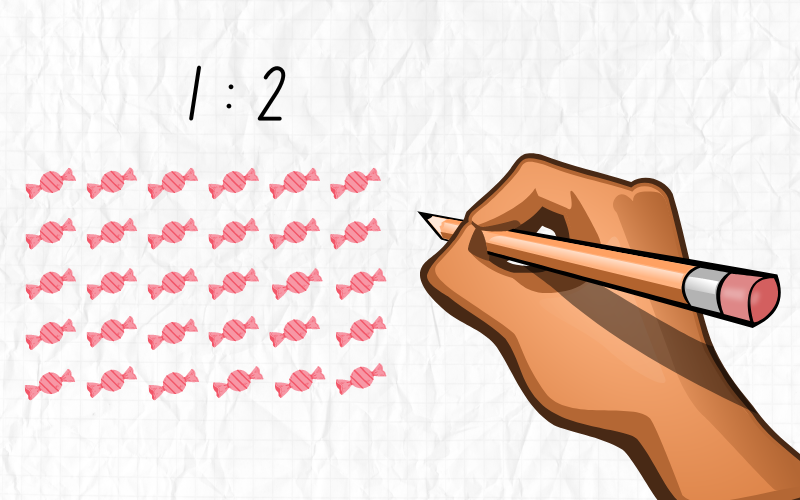
Alan and Albert have 30 sweets. They are going to share the sweets, but Albert paid more for them than Alan, so they have decided to share them in the ration 1:2.
How many sweets did Albert get?
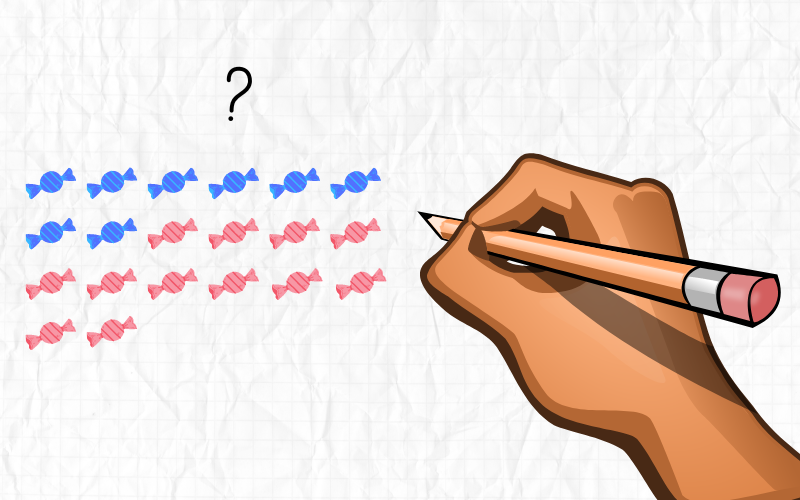
In a bag of 20 sweets, there are 8 blue sweets and 12 pink sweets. What is the ratio of blue to pink sweets?
If you need to prepare for a number of different employment tests and want to outsmart the competition, choose a Premium Membership from JobTestPrep.
You will get access to three PrepPacks of your choice, from a database that covers all the major test providers and employers and tailored profession packs.
Prepare for Any Job Assessment Test with JobTestPrep

How should the scale factor of 3 cm : 15 m be expressed as a simplified ratio?

Simplify 10:2.5

If you go to the shop and buy 4 apples for £0.64, how much would 11 apples cost?

Andrew and James have 400 sweets and they need to share them in the ratio 5:3. How many sweets does each of them receive?
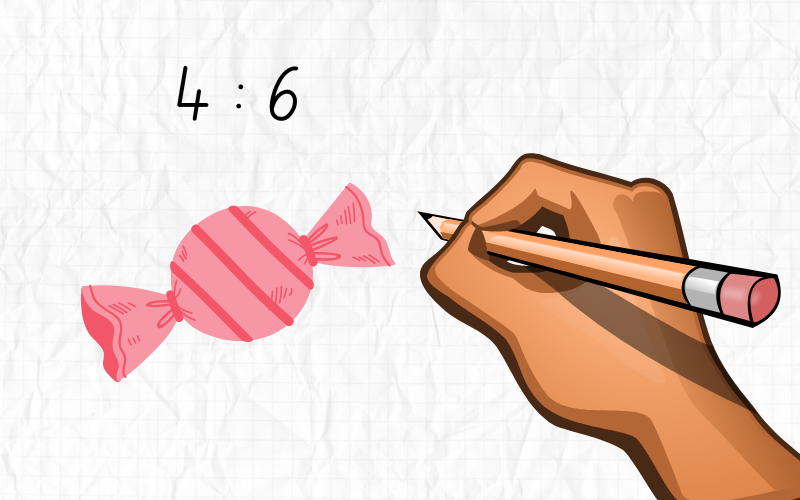
The sweet company likes to put uneven numbers of sweets in their bags. They are currently creating a bag of blue and pink sweets in the ratio 4:6.
If you get a bag with 12 blue sweets in it, how many will there be in total?
In a fruit bowl, there are apples, oranges, and bananas.
If there are 4 apples, 6 oranges, and 12 bananas, what is the ratio of the fruit?
Prepare for Any Job Assessment Test with JobTestPrep
Common Mistakes to Avoid When Learning How to Calculate Ratios
- Don’t misread the information. Sometimes the way a question is worded can make it difficult to get the ratio round the right way, and displaced numbers will make your entire calculation incorrect.
- Make sure you know what the question is asking for. Sometimes it might seem that you need to make a calculation based on the parts, when you actually need to use all the numbers. For example, the question might be about finding the proportion of one thing against the total number of things.
- Ratios are always whole numbers, not decimals or fractions.
- Always present your ratios fully simplified.
The formula you use for calculating ratios is a:b = a/b
For example, the ratio of a and b is 3:5.
You know that a = 86 and you need to find b.
To calculate the ratio you would take the following steps:
a:b = 3:5
a/b = 3/5
86/b = 3/5
b = (5/3) x 86
b = 143.3
Depending on the information you have, the easiest way to calculate a ratio is:
Scenario A: What is 3:5 of $30?
- Find the total number of parts – if the ratio is 3:2, the total is 5
- Divide the figure by the number of parts to find the sum of one part – $30 divided by 5 = 6. One part is 6
- Multiply each number in the ratio by the value of one part – 3 x 6 and 2 x 6. If you are finding 3:2 of $30 your answer is 18:12
Scenario B: What is the ratio of apples to lemons in a field of 100 apples and 80 lemons?
- Find your two starting figures. For example, if you were looking for the ratio of apples to lemons in a field that has 100 orchids and 80 tulips. 100 and 80 are your starting figures.
- In this scenario you are trying to find the simplest ratio. You do this by finding the highest number that both figures divide into. In this case, 20 is the highest.
- You then divide each figure by this number: 100/20 = 5 and 80/20 = 4
- The answers give you your ratio of 5:4
Ratio analysis is an analytical technique that combines several financial ratios to assess a company’s financial position.
Depending on the figures you are asked to find, you might use one, some, or all of these ratios:
Liquidity
- Current Ratio = Current Assets / Current Liabilities
- Cash Ratio = Cash & Cash Equivalents / Current Liabilities
- Quick Ratio = (Cash & Cash Equivalents + Accounts Receivables) / Current Liabilities
Solvency
- Debt-To-Equity Ratio = Total Debt / Total Equity
- Debt Ratio = Total Debt / Total Assets
- Interest Coverage Ratio = EBITDA / Interest Expense
Efficiency
- Receivables Turnover Ratio = Sales / Accounts Receivable
- Inventory Turnover Ratio = COGS / Inventories
- Payable Turnover Ratio = COGS / Accounts Payable
- Asset Turnover Ratio = Sales / Total Assets
- Net Fixed Asset Turnover Ratio = Sales / Net Fixed Assets
- Equity Turnover Ratio = Sales / Total Equity
Profit
- Gross Margin = (Sales – COGS) / Sales
- Operating Profit Margin = EBIT / Sales
- Net Margin = Net Income / Sales
- Return on Total Asset (ROA) = EBIT / Total Assets
- Return on Total Equity (ROE) = Net Income / Total Equity
To calculate these ratios, you simply need to enter the correct figures or enter these formulas into a program such as Microsoft Excel.
You can find a ratio’s simplest form by finding a number both sides of the ratio have in common and dividing them.
For example, a ratio of 20:60.
Both sides are multiples of 10.
20/10 = 2
60/10 = 6
The ratio then becomes 2:6
Or if you wanted to make it simpler both 2 and 6 are multiples of 2.
2/2 = 1
6/2= 3
The final ratio is 1:3.
To calculate ratio analysis there are several programs and apps you can use.
These include:
- Ready Ratios
- Microsoft Excel
- Google Sheets
- Financial Ratio Calculator app
If you want to find the ratio of two numbers online you would use a ratio calculator such as the Calculator Soup.
There are specific online calculators for ratios, but is it also possible to use a physical calculator to calculate your ratios.
The method you use to find ratios on a calculator differs depending on the information you have. However, you will follow the same easy steps you would use if you were to mentally calculate:
- Find your two starting figures. For example, if you were looking for the ratio of orchids to tulips in a garden that has 150 orchids and 70 tulips. 150 and 70 are your starting figures.
- In this scenario you are trying to find the simplest ratio. You do this by finding the highest number that both figures divide into. In this case, 10 is the highest.
- Using your calculator, enter the first number and divide by the number you found: 150/10 = 15 and 70/10 = 7
- The answer to both is your ratio: 15:7
Alternatively, if you wanted to know the ratio of a figure, say 6:2 of $70, you would:
- Determine the total number of parts: if the ratio is 6:2, the total is 8
- Divide the figure by the number of parts to find the sum of one part: $70 divided by 8 = 8.75. So one part is 8.75.
- Multiply each number in the ratio by the value of one part: 6 x 8.75 and 2 x 8.75
Therefore 6:2 of $70 is 52.5:17.5
Summary
Ratios are a mathematical expression to compare units.
They can be used as equivalent ratios to help you scale up numbers – for example, quantities of ingredients for baking a cake.
In mathematical terms, they can be used to work out problems relating to direct proportion, where the increase or decrease in units occurs in the same ratio.
Ratios can be simplified and, in most cases, it is preferable to give a simplified ratio as an answer. Like fractions, you can simplify a ratio by dividing it by the highest common factor.
When using scales on drawings or models, ratios help to describe the relationship between the real-life item and the created one – allowing for accurate measurements as well as an idea of proportion.
When trying to understand ratios, it is easiest to work with the same units.
Remember, to fully explore a ratio, you need to use a whole number, so try to avoid creating any decimals when you are transforming units to match.
Practicing ratio problems will make them much easier to understand.
It is likely you will use ratios throughout your life and might be tested on math skills like these when applying for jobs in technical industries.

